Tangente (trigonometría)

Tangente
Gráfica de Tangente
Definición	tg x
Dominio	${\displaystyle \mathbb {R} \setminus \left\{{\frac {\pi }{2}}+n\pi \right\},\;n\in \mathbb {Z} }$
Imagen	${\displaystyle \mathbb {R} }$
Cálculo infinitesimal
Derivada	${\displaystyle \sec ^{2}x}$
Función inversa	arctan x
[editar datos en Wikidata]

En matemática, la tangente es una función impar y es una función periódica de periodo ${\displaystyle \pi }$ con indeterminaciones en ${\displaystyle {\frac {\pi }{2}}+n\pi ,\;n\in \mathbb {Z} }$, y además una función trascendente de variable real. Su nombre se abrevia de las dos siguientes formas: tan y tg.^[1]​

${\displaystyle \operatorname {tg} \;x=-\operatorname {tg} (-x)}$

${\displaystyle \operatorname {tg} \;x=\operatorname {tg} (\pi +x)}$

En trigonometría, la tangente de un ángulo (de un triángulo rectángulo) se define como la razón entre el cateto opuesto y el adyacente:

${\displaystyle \operatorname {tg} \alpha ={\frac {a}{b}}={\frac {BC}{OC}}}$

Esta razón no depende del tamaño del triángulo rectángulo escogido sino que es una función dependiente del ángulo ${\displaystyle \alpha .}$

Esta construcción permite representar el valor del tangente para ángulos no agudos.

Semejanza

Dada la circunferencia de radio 1 y una recta r que pasa por el centro, describe un triángulo rectángulo con ángulo ${\displaystyle \alpha }$ como en la imagen, y tenemos las siguientes relaciones por semejanzas:

${\displaystyle \operatorname {tg} \alpha ={\frac {CB}{AC}}={\frac {DE}{AD}}={\frac {DE}{1}}=DE}$

El segmento ${\displaystyle DE}$ representa el valor de la tangente de ${\displaystyle \alpha .}$

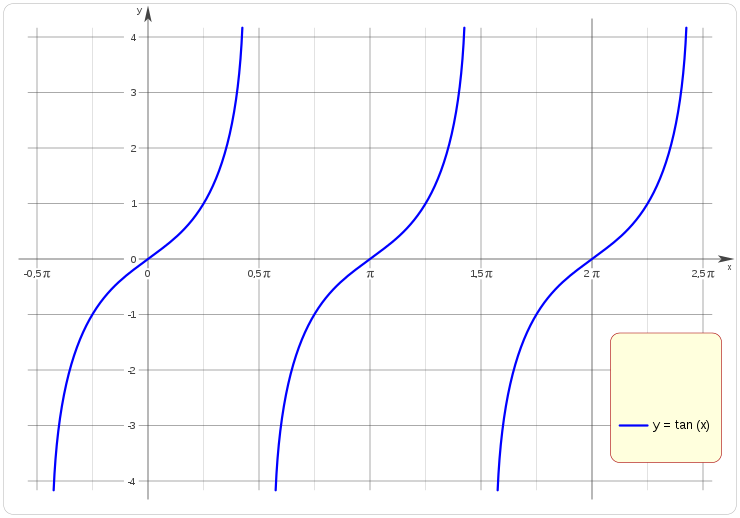
Representación gráfica

Identidades

Tangente de la suma de dos ángulos

Esta identidad trigonométrica parte de la identidad de la suma de dos ángulos ya conocida para el seno y el coseno.

• Dados los ángulos ${\displaystyle \phi ,\theta \ }$:

${\displaystyle \operatorname {tg} \left(\phi +\theta \right)={\cfrac {\operatorname {sen}(\phi +\theta )}{\cos(\phi +\theta )}}}$

• Reemplazando por las identidades antes mencionadas:

${\displaystyle \operatorname {tg} \left(\phi +\theta \right)={\cfrac {\operatorname {sen} \phi \cos \theta +\cos \phi \operatorname {sen} \theta }{\cos \phi \cos \theta -\operatorname {sen} \phi \operatorname {sen} \theta }}}$

• Dividiendo al numerador y al denominador por ${\displaystyle \cos \phi \cos \theta \,}$:

${\displaystyle \operatorname {tg} \left(\phi +\theta \right)={\cfrac {\cfrac {\operatorname {sen} \phi \cos \theta +\cos \phi \operatorname {sen} \theta }{\cos \phi \cos \theta }}{\cfrac {\cos \phi \cos \theta -\operatorname {sen} \phi \operatorname {sen} \theta }{\cos \phi \cos \theta }}}}$

• Separando la suma y la resta y simplificando:

${\displaystyle \operatorname {tg} \left(\phi +\theta \right)={\cfrac {\operatorname {tg} \phi +\operatorname {tg} \theta }{1-\operatorname {tg} \phi \operatorname {tg} \theta }}}$

Tangente de la diferencia de dos ángulos

• ${\displaystyle \operatorname {tg} \left(\phi +(-\theta )\right)={\frac {\operatorname {tg} \phi +\operatorname {tg} (-\theta )}{1-\operatorname {tg} \phi \operatorname {tg} (-\theta )}}}$

• Al ser una función impar, se obtiene:

${\displaystyle \operatorname {tg} \left(\phi -\theta \right)={\frac {\operatorname {tg} \phi -\operatorname {tg} \theta }{1+\operatorname {tg} \phi \operatorname {tg} \theta }}}$

Fórmula resumida

${\displaystyle \operatorname {tg} \left(\phi \pm \theta \right)={\frac {\operatorname {tg} \phi \pm \operatorname {tg} \theta }{1\mp \operatorname {tg} \phi \operatorname {tg} \theta }}}$

Tangente del ángulo doble

Partiendo de

${\displaystyle \operatorname {tg} \left(\phi +\theta \right)={\frac {\operatorname {tg} \phi +\operatorname {tg} \theta }{1-\operatorname {tg} \phi \operatorname {tg} \theta }}}$

y haciendo ${\displaystyle \phi =\theta \,}$ entonces:

${\displaystyle \operatorname {tg} \left(2\phi \right)={\frac {2\operatorname {tg} \phi }{1-\operatorname {tg} ^{2}\phi }}}$

Tangente del ángulo triple

Conociendo la tangente del ángulo ψ, hallar la tangente de 3ψ

${\displaystyle \operatorname {tg} \left(3\psi \right)={\frac {3\operatorname {tg} \psi -\operatorname {tg} ^{3}\psi }{1-3\operatorname {tg} ^{2}\psi }}}$

Tangente del ángulo mitad

Se trata de hallar la tangente de la mitad de θ, conociendo los de θ:

${\displaystyle \operatorname {tg} {\frac {\theta }{2}}={\frac {\operatorname {sen} \theta }{1+\cos \theta }}}$ ^[2]​

Derivada de la tangente

${\displaystyle [\operatorname {tg} (x)]'=\sec ^{2}(x)\,}$

Véase también

• Función impar
• Trigonometría

Referencias y notas

Enlaces externos

• Weisstein, Eric W. «Tangente». En Weisstein, Eric W, ed. MathWorld (en inglés). Wolfram Research.